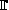 p. The order of P
is the smallest positive integer n such that P(x) divides xn-1.
n is also equal to the multiplicative order of any root of P. It is a
divisor of pd-1. P is a primitive polynomial if
n=pd-1.
p. The order of P
is the smallest positive integer n such that P(x) divides xn-1.
n is also equal to the multiplicative order of any root of P. It is a
divisor of pd-1. P is a primitive polynomial if
n=pd-1.
This tool allows you to enter a polynomial and compute its order. If you enter a reducible polynomial, the orders of all its non-linear factors will be computed and presented.